
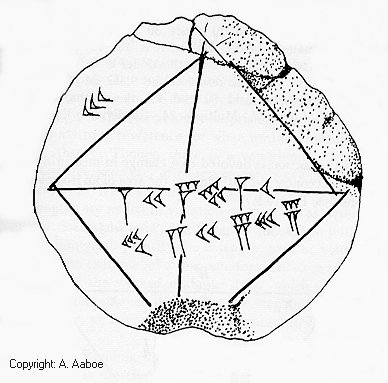
This tablet is a round school tablet of unknown provenance from the Old Babylonian period. It has a picture of a square with both diagonals drawn in. On one side of the square is written the number 30, along one of the diagonals is the number 1,24,51,10 and below it is 42,25,35.
It is easy to see that 30 times 1,24,51,10 is 42,25,35 (or, recalling that the reciprocal of 30 is 2, that 42,25,35 times 2 is 1,24,51,10). From the positioning of the numbers, the natural interpretation to make is that a square with side of length 30 (or 1/2) has diagonal of length 42,25,35. This means that the number 1,24,51,10 must be the 'coefficient of the diagonal of a square' and, indeed we do have an Old Babylonian coefficient list that has this number (see MCT text Ue (YBC 7243)).
We know that the ratio of the side to diagonal in a square is 1 to the square root of 2. Since root(2) is irrational, it cannot be expressed as a finite sexagesimal number, so 1,24,51,10 can only be approximate. In fact, the square of 1,24,51,10 is 1,59,59,59,38,1,40, a remarkably good approximation to 2
Why choose a side of 30 in a school exercise on diagonals? Because it is the only choice that makes the diagonal of the square (42,25,35) equal to the reciprocal of the coefficient (1,24,51,10) That is, 1,24,51,10 is root(2) and 42,25,35 is 1/root(2). Given the importance the Babylonians attached to reciprocals, this can hardly be a coincidence. It is a nice exercise in algebra to see why you want a square of side 1/2 rather than 1.
For a recent analysis of how Mesopotamian scribes might have
determined
the approximation used, see Fowler, D.H. and Robson, E.R. (1998).
'Square
root approximations in Old Babylonian mathematics: YBC 7289 in
context.'
Historia Mathematica 25, 366-378.
Some excellent large-size photographs of the tablet
along with some commentary and analysis are available on Bill
Casselman's website. I encourage you to visit there.
Go to Mesopotamian
Mathematics.
Last modified: 18 September 2006
Duncan J. Melville